Introduction: A Complex number is a part of Mathematics that does not have any fixed value. The issue with this section is that people can’t find the actual value. Despite this feature, you get to use it in all schemes and problems. The chapter on vectors in Physics relies on this topic as well. Students have to plot graphical notations to find the direction of vectors. When you open your textbook, you will see that the question is in “x+iy” form. The “x” section is what Mathematician’s term as the real part. “y” is the imaginary value attached to the equation.

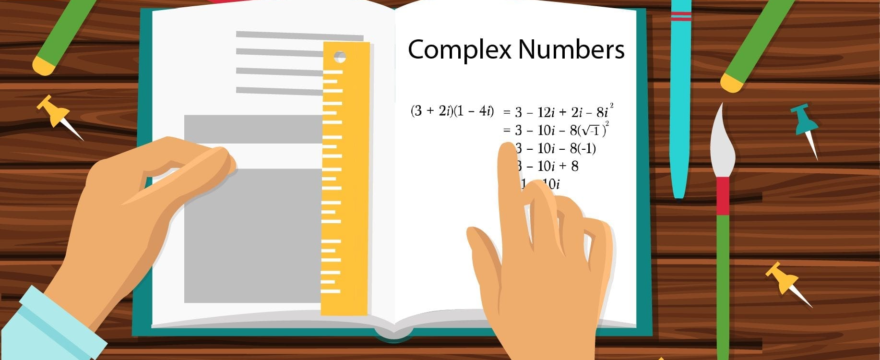
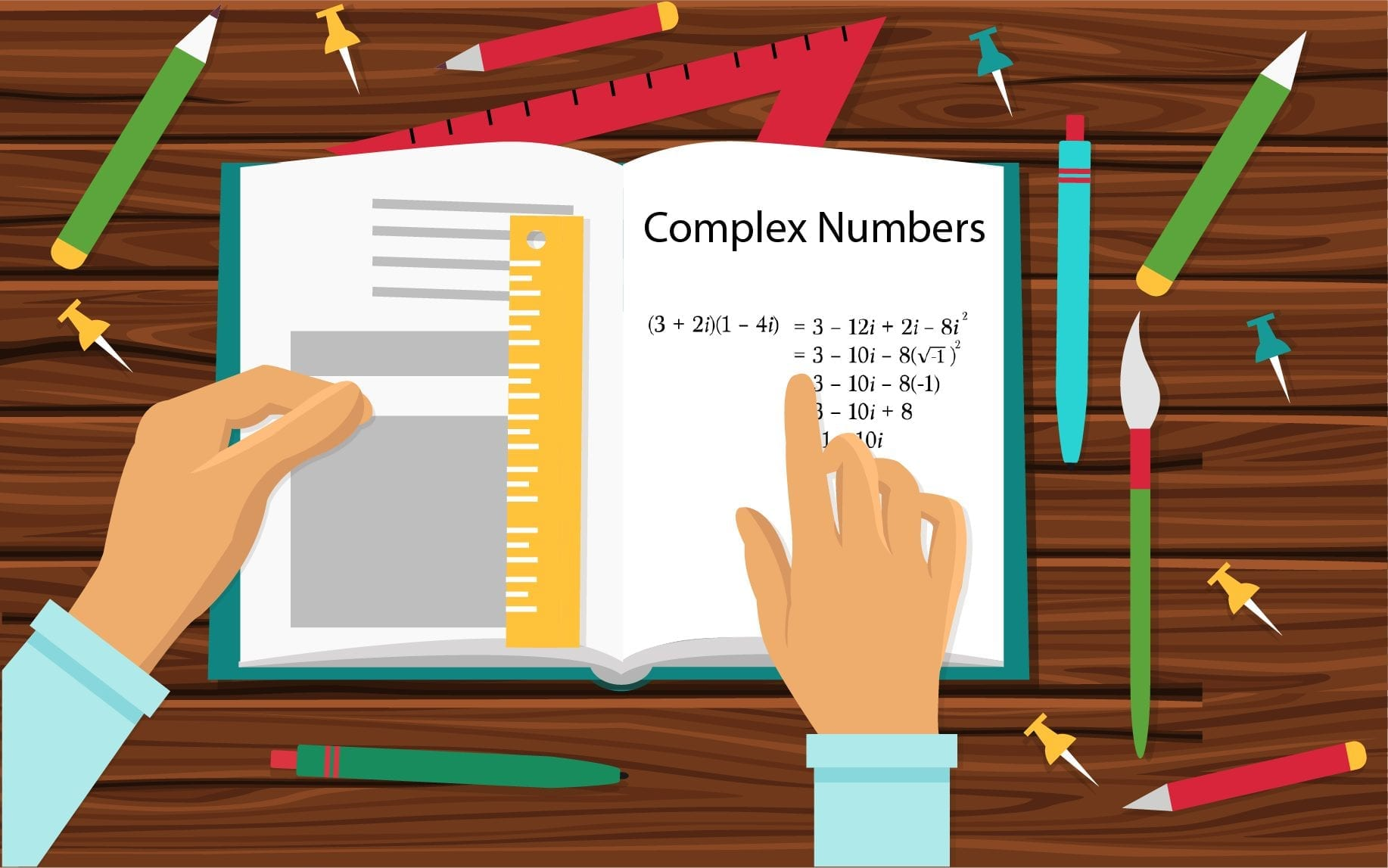
The idea of a complex number: As the name suggests, “i” is a short form of imaginary. It has a definite value that is equal to root over -1. Mathematicians know that this value has no existence in our reality. But that does not mean that the relevance of this point is zero. Solving certain equations will mean that the imaginary part is required. This is the eye-opening part of the complex number chapter students usually study. Apply the rules carefully and you can proceed to the next stage of the equations.

When are they equal?

Looking at integers and determining equality is simple. The sign and value are all that a student needs to match. But in complex numbers, the algorithm is a bit unique. Both the real and imaginary boxes carry the same weightage. A student must know the tricks behind comparing both these sections. Only then the solution can be found and you can tell if it is equal. The coefficient attached to “i” is termed imaginary. If the solution is xi it means that x multiplied by i is the imaginary value.

Real or imaginary complex numbers: This entire procedure revolves around just the imaginary portion. A prime feature of this chapter is that real numbers have no “i”. Any real number such as “a” can be depicted as “a+0i”. To make it real the imaginary side needs to be negligible or vanished. Perform the equation to see what you get for both parts. The solution is enough to determine the reality of a number. Many complex integers exist as purely the imaginary form. For them, the real part has no existence or importance. It becomes equal to the value that zero has. You will see it just as a multiple of the symbol “i”.

How to operate with complex numbers?

Adding two complex numbers follows a bit of a different rule. Understanding the structure and form of these equations is very crucial. Every complex number contains two major parts as per the chapter. To do any operation such as addition or subtraction, consider both these parts equally. The final solution will have both real and imaginary sections as well. Add the real parts of the question present in the sheet. Then follow the same rule on the imaginary sections as well. Combine both of them with the symbol “i” and get the complex answer.

Representing the graph: Graphical representation is one of the most common uses of complex equations. It allows you to understand different concepts that cannot be written. Plot the real part in the x-axis to get a point. This will be present on the bottom side of the graphical solution. Then just attach the coefficient of “i” to the y-axis. You will get the graph that your examiner will demand from you.

Complex numbers have multiple properties which are almost similar to integers. You can get a subscription to the Cuemath website and explore these interesting chapters. You can directly converse with teachers who have years of experience in these topics.